30) For this question, we are given an acceleration graph for two objects and we want to find the speed and distance covered by the two objects during the same time period. First, the integral of the acceleration graph can be described as the change in velocity from time t = 0, to t = T. This means we can solve for the velocity at time t = T.
Now, taking a look at the graph, we can find the velocity at time t = T.
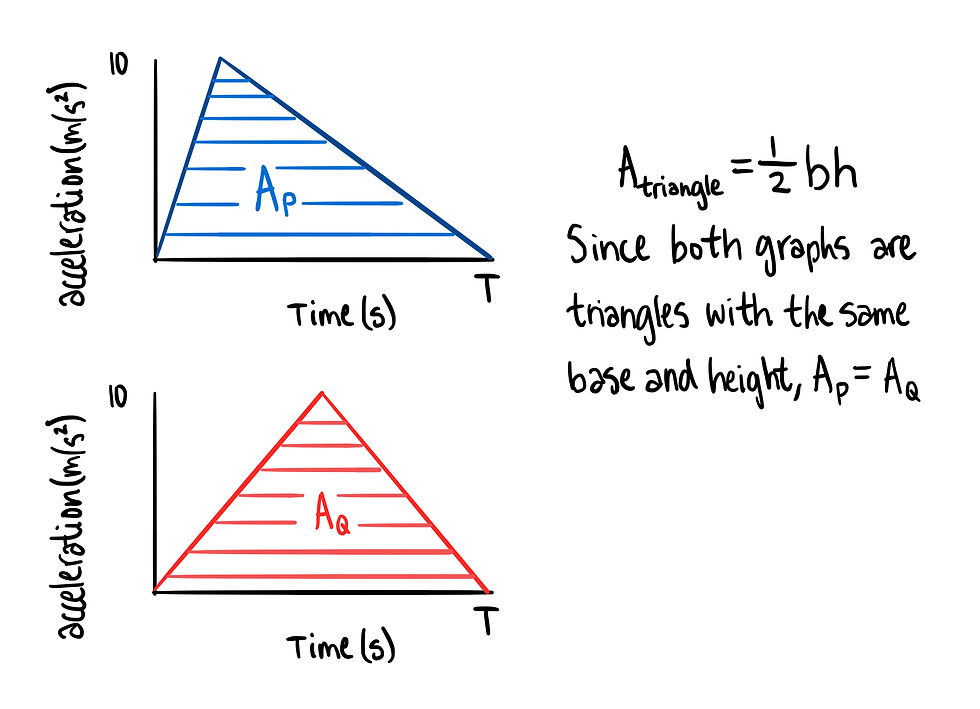
Since both graphs have the same integral, they have the same velocity at time t = T.
Next, we need to find the relation between the position of the two objects. The two objects start at the same point, but the velocity of car P is greater than the velocity of car Q for every time except t = 0 and t = T. This means that car P has a greater average velocity that car Q, so car P travels a further distance and ends up in a position x[p] > x[q].
Answer: A
Comments