14) We want to find out which satellite's speed it greater. To solve this problem, we can use equations for centripetal force and for universal gravitational force. Centripetal force is not itself a force, but a term that describes a force directed to the center of the object’s orbit. Gravitational force is the force that pulls one mass to another.
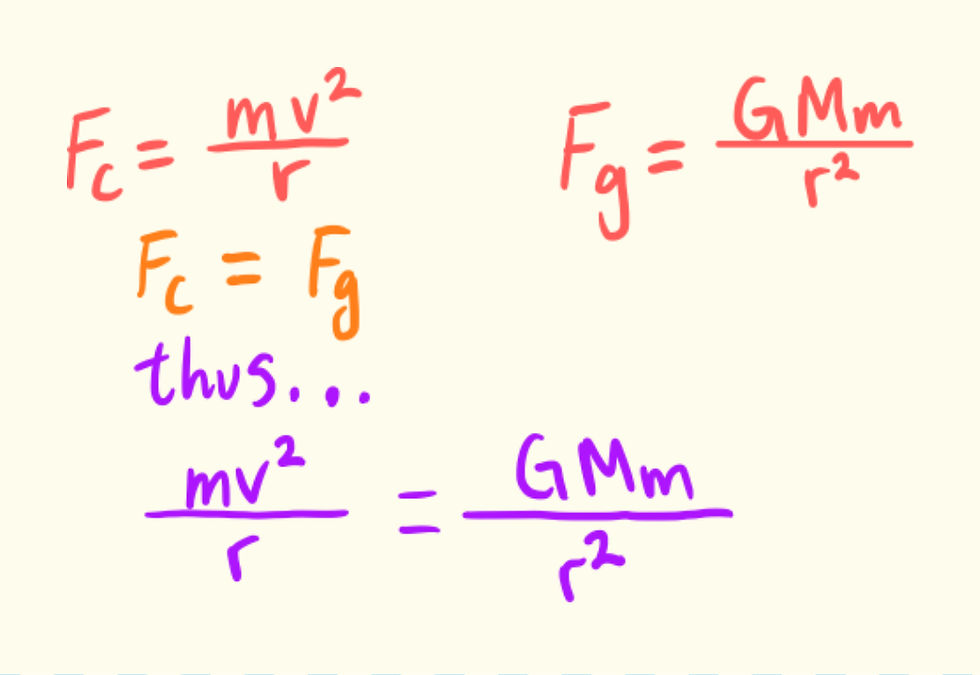
Since the question is asking about the relationship of the satellites' speeds, we should simplify and solve for speed.
After solving for speed, it is easily noticeable that the mass of the orbiting object is irrelevant and that information is provided to trick you. The question doesn't call for the exact values just the ratio, so we can further simplify this by making a proportion between speed and distance and ignoring any constants or coefficients. The only constants or coefficients are G and M.
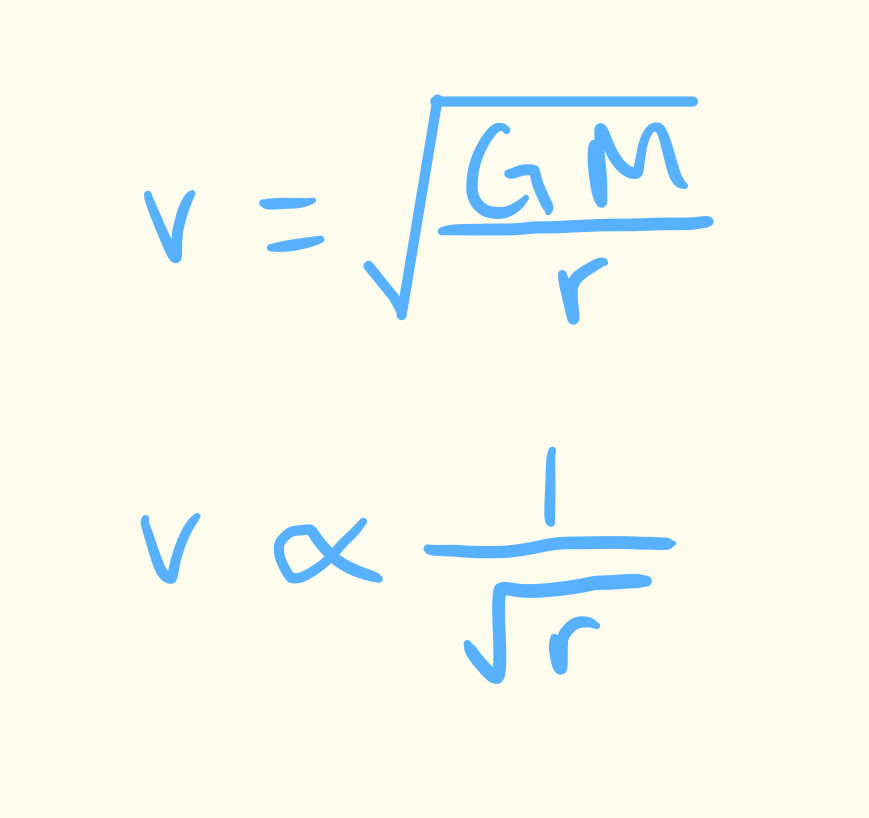
With this proportion, it is easy to tell that speed is inversely related to the square root of distance. Finally, if we plug in r for satellite #1 and 2r for satellite #2, this is what we will get...
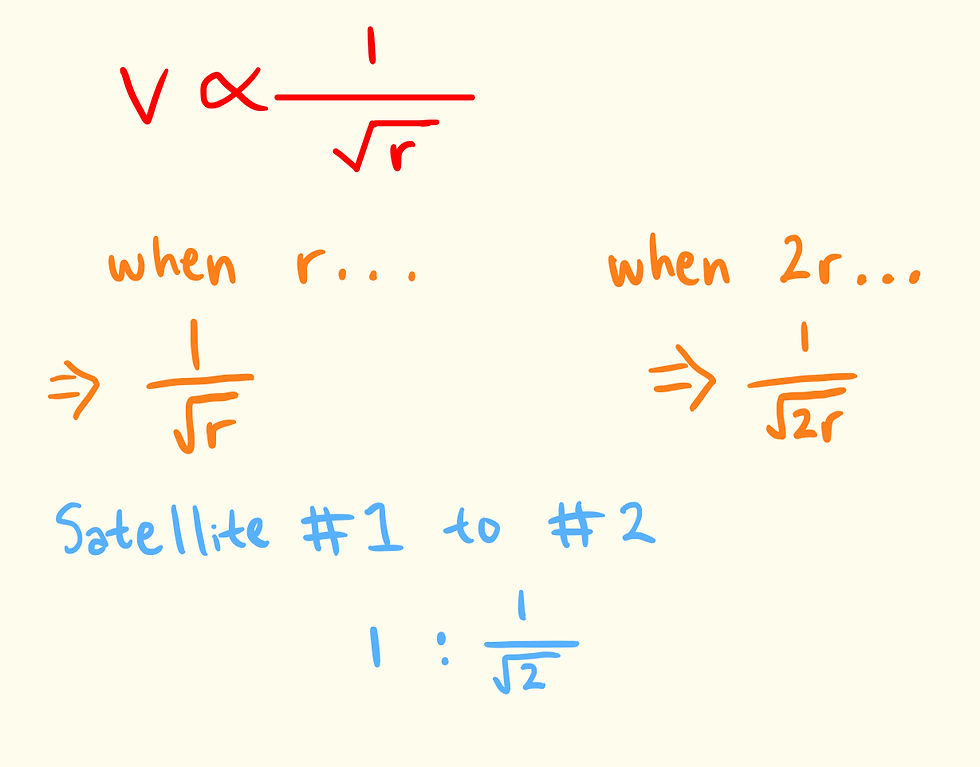
Comparing these values, we can see that satellite #2 has a slower speed by a factor of square root 2. This means satellite #1 will have a faster speed by a factor of square root 2.
Answer: A
Comments